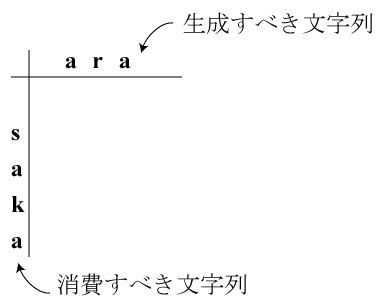
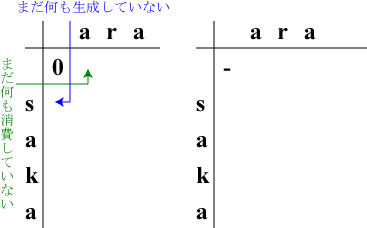
| 欄 | 操作 | 編集中の文字列 |
|---|---|---|
| (0,0) | 初期状態 | |saka |
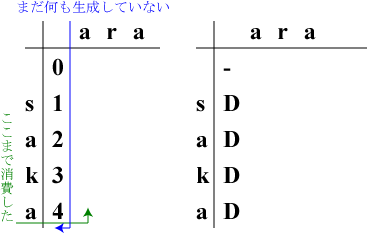
| 欄 | 操作 | 編集中の文字列 |
|---|---|---|
| (0,0) | 初期状態 | |saka |
| (1,0) | 's'を『削除』 | |aka |
| (2,0) | 'a'を『削除』 | |ka |
| (3,0) | 'k'を『削除』 | |a |
| (4,0) | 'a'を『削除』 | | |
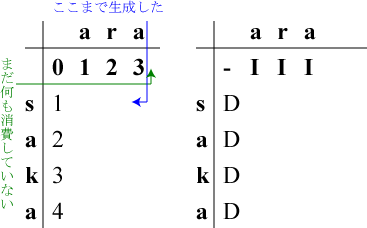
| 欄 | 操作 | 編集中の文字列 |
|---|---|---|
| (0,0) | 初期状態 | |saka |
| (0,1) | 'a'を『挿入』 | a|saka |
| (0,2) | 'r'を『挿入』 | ar|saka |
| (0,3) | 'a'を『挿入』 | ara|saka |
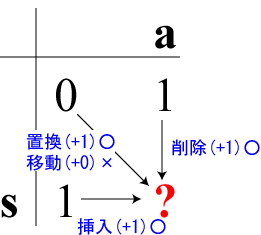
- (L-1,R-1)の状態からの「置換」
- (L-1,R-1)の状態からの「カーソル移動」←文字が等しい場合だけ
- (L-1,R)の状態からの「削除」
- (L,R-1)の状態からの「挿入」
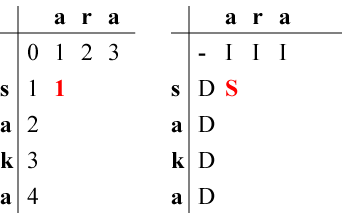
- 「(0,0)の状態から's'を'a'に『置換』」、コストは 0+1=1
- 「以前に'a'を『挿入』した(0,1)の状態からの's'の『削除』」、コストは1+1=2
- 「以前に's'を『削除』した(1,0)の状態からの'a'の『挿入』」、コストは1+1=2
| 欄 | 操作 | 編集中の文字列 |
|---|---|---|
| (0,0) | 初期状態 | |saka |
| (1,1) | 's'を'a'に『置換』 | a|aka |
欄(1,1)の状態は、操作の順番はともかく 「『消費すべき文字列』から先頭の's'が無くなり、 『生成すべき文字列』から先頭の'a'が無くなった」状態です。 その状態にたどり着くための編集操作の最小コストが1であったわけです。
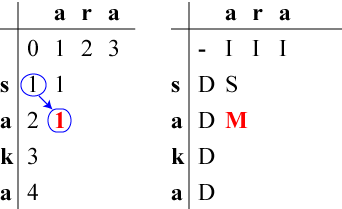
| 欄 | 操作 | 編集中の文字列 |
|---|---|---|
| (1,0) | 's'を『削除』 | |aka |
| (2,1) | 同じ'a'なので『カーソル移動』 | a|ka |
欄(2,1)の状態は、「まず『消費すべき文字列』から先頭の's'を『削除』し、 その次に『消費すべき文字列』と『生成すべき文字列』がともに'a'であったので 『カーソル移動』した」状態です。 その状態にたどり着くための演習操作の最小コストが「削除(コスト1)」と 「カーソル移動(コスト0)」の合計で、1です。
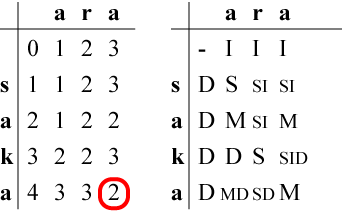
表を全て記入した状態です。
右表の中で複数の記号が併記されている場合はどちらの操作でも 最小コストになることを表しています。 たとえば"SI"という記号は「置換または挿入のどちらの操作でもOK」 の意味です。
左表の最右下の欄である(4,3)を見ることで最小コストは2であることがわかります。
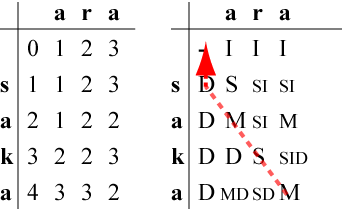
- SかMならば置換かカーソル移動によってその欄に到達したので、左上へ
- Iならば挿入によってその欄に到達したので、左へ
- Dならば削除されてその欄に到達したので、上へ
最小コストの操作手順は以下のようになります。
| 欄 | 操作 | 編集中の文字列 |
|---|---|---|
| (0,0) | 初期状態 | |saka |
| (1,0) | 's'を『削除』 | |aka |
| (2,1) | 'a'は『カーソル移動』 | a|ka |
| (3,2) | 'k'を'r'に『置換』 | ar|a |
| (4,3) | 'a'は『カーソル移動』 | ara| |